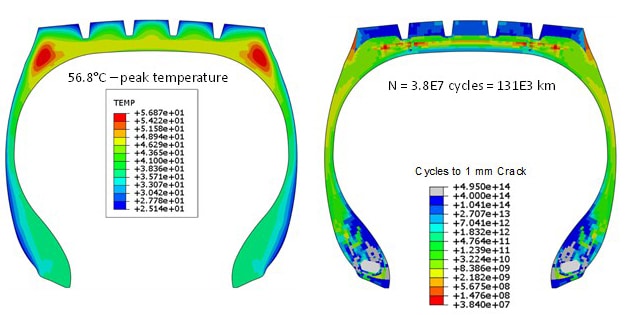
Every year, the top minds from academia, government and industry gather in Akron to share their work at the Tire Society annual meeting, and to... Continue reading
Return to previous page